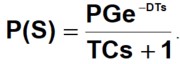Home About us Contact us Protuner Loop Analyser & Tuner Educational PDFs Loop Signatures Case Histories
Michael Brown Control Engineering CC
Practical Process Control Training & Loop Optimisation
LOOP SIGNATURE 25
TUNING - PART 3
RESULTS OF TUNING A PARTICULAR SIMPLE SELF-REGULATING PROCESS
BY SEVERAL DIFFERENT METHODS
A couple of SWAG methods of tuning were given in the previous Loop Signature article. I have tuned a simple self-regulating process using those methods, as well as two other tuning methods, one of them being the sophisticated Protuner tuning package, which is the system I employ. The results are compared below. (The tests were performed on a very accurate and powerful simulation package).
The process that was tuned is a simple first order lag, deadtime, self-regulating process, with a model:
Where: PG = 1.5.
DT = 3.0 seconds.
And TC = 30.0 seconds.
Note that this model meets all the SWAG model tuning assumptions listed in the previous Loop Signature article. It would have been terribly easy for me to have used a more complex model, and then to have said that SWAG tuning methods are useless. Instead they should work extremely well on this model.
For interest sake, the Ziegler Nichols ultimate sensitivity tuning test gave an ultimate gain of 10.45, and an ultimate period of 11.5 seconds. With these figures and the figures given above for the model, the reader should be able to calculate tuning for the Ziegler Nichols, the Lambda, and for the IMC methods using the formulae given in the last article. The results obtained should correspond to those listed in the table below.
First a word about the other two tuning types shown in the table that have not been previously discussed:
The SWAG method proposed by Cohen and Coon appears in many articles on tuning, and also in a few manufacturers’ controller manuals. I have not given the formula, which are extremely long and laborious, and also because in my experience the method is not at all good, and seldom gives even reasonable tuning.
The Protuner, which is a commercially available loop analytical package, contains a tuning system developed by a particularly brilliant control engineer, David Ender of Techmation Inc., in conjunction with an eminent mathematician, Dr Alin Popluca. The tuning is accomplished by translating a step response into the frequency domain, which then allows the true theory as developed by the early mathematicians in the 1930’s, to be used to calculate tuning for values for any desired response. On top of that, the software also contains a “tree of knowledge” based on Mr Ender’s experience obtained by optimising many thousands of control loops during his career. This also refines the tuning for certain particular more difficult types of processes, for example dead-time dominant processes.
The beauty of the system is that it is not a SWAG method; it does not try to obtain tuning from a model, but uses the frequency response to obtain the tuning. Over the many years I have been using the Protuner I have come to rely absolutely on its ability to tune any type of process dynamic. In fact it is the only tuning system of the many that I have tried that consistently gives good results on any type of process dynamics. Provided the step test gives a true representation of the process, the Protuner gives excellent and very reliable tuning.
As can be seen in the table, the tunings obtained by the various methods differ quite widely in general. The Protuner’s result should in fact be different, as its fast tune allows for one overshoot and undershoot in the response to a setpoint step change. All of the others should have been the same as they all try and give a quarter wave damped response. (See previous Loop Signature article for the reasons we do not like this response). Delegates on my courses always ask why the different tunings are so different? The answer lies in the fact that no SWAG method really works very well. If any of them had been able to give correct tuning, then the matter would have stopped there. There would not have been a proliferation of tuning methods that we see today. Literally hundreds of people have, and still are trying to come up with better and more consistent SWAG methods.
Let us examine how each of the tunings reacted to a step response of setpoint:
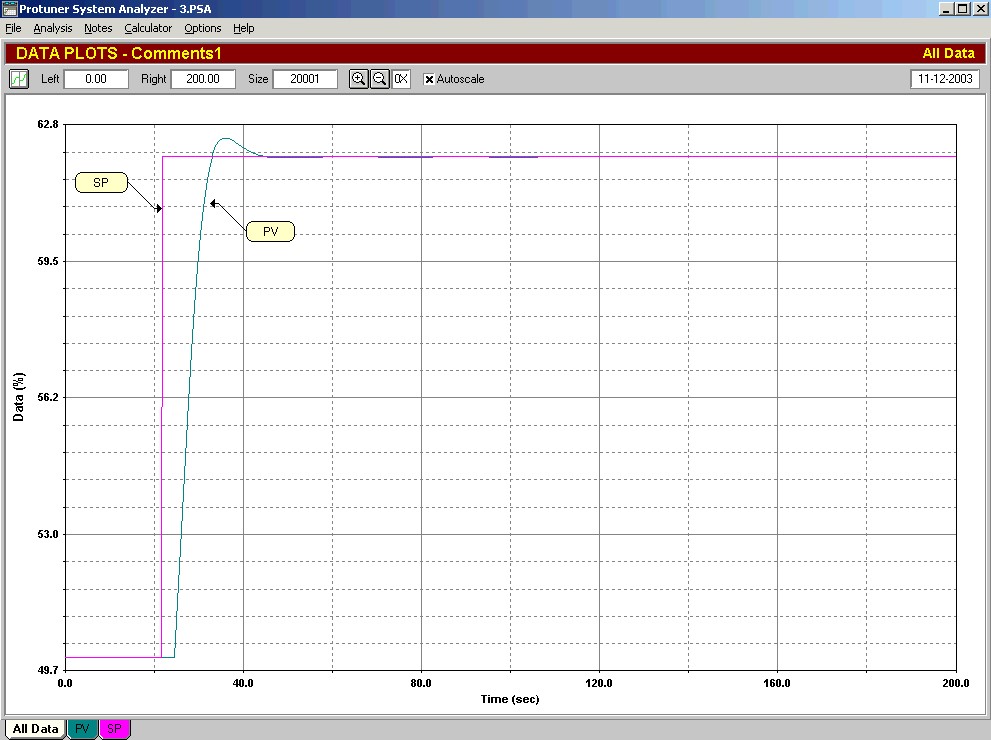
Figure 1
Figure 1 is the response given by the Ziegler Nichols ultimate sensitivity tuning method. It’s actually pretty good. It is pretty close to quarter wave damping, which people might find hard to believe, but as mentioned in the previous article, its actually very difficult to see four cycles in a true quarter wave damped response.
Figure 2
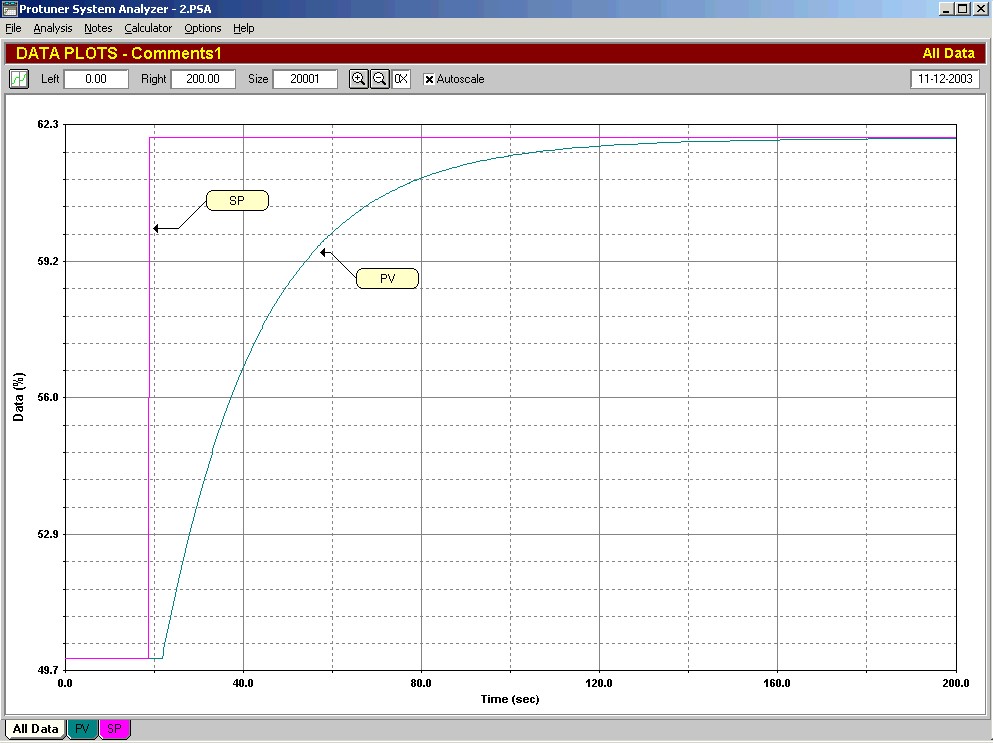
Figure 2 is the Lambda method. It is far from quarter wave damping, and is in fact very over-damped.
Figure 3
Figure 3 is the IMC tuning. It is much better; but is still quite far away from quarter wave damping.
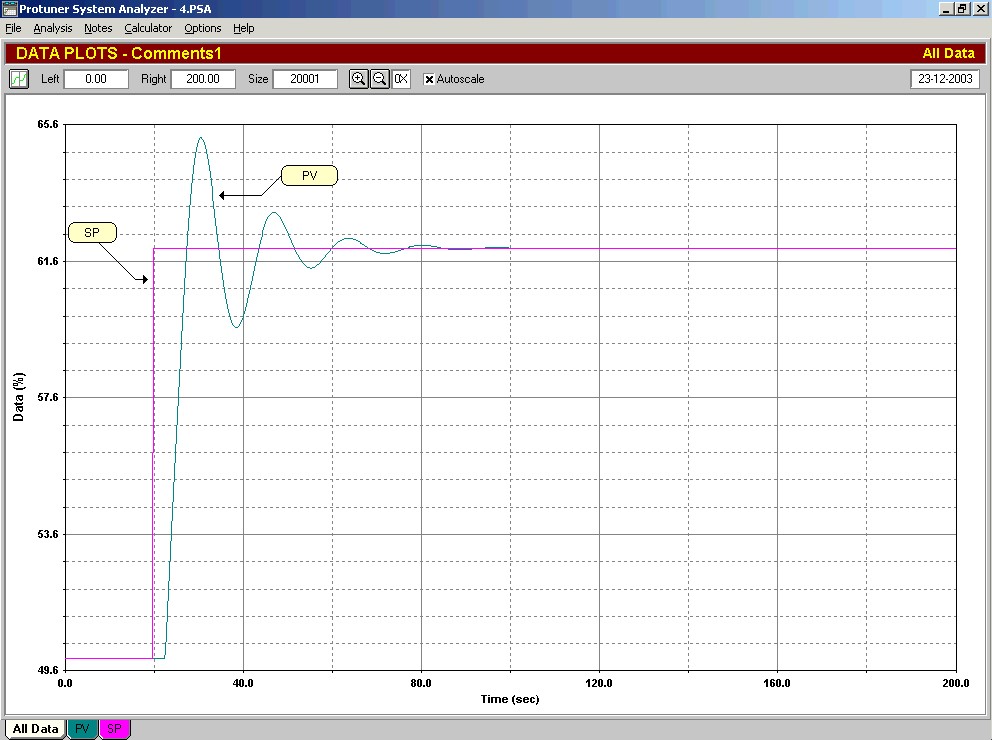
Figure 4
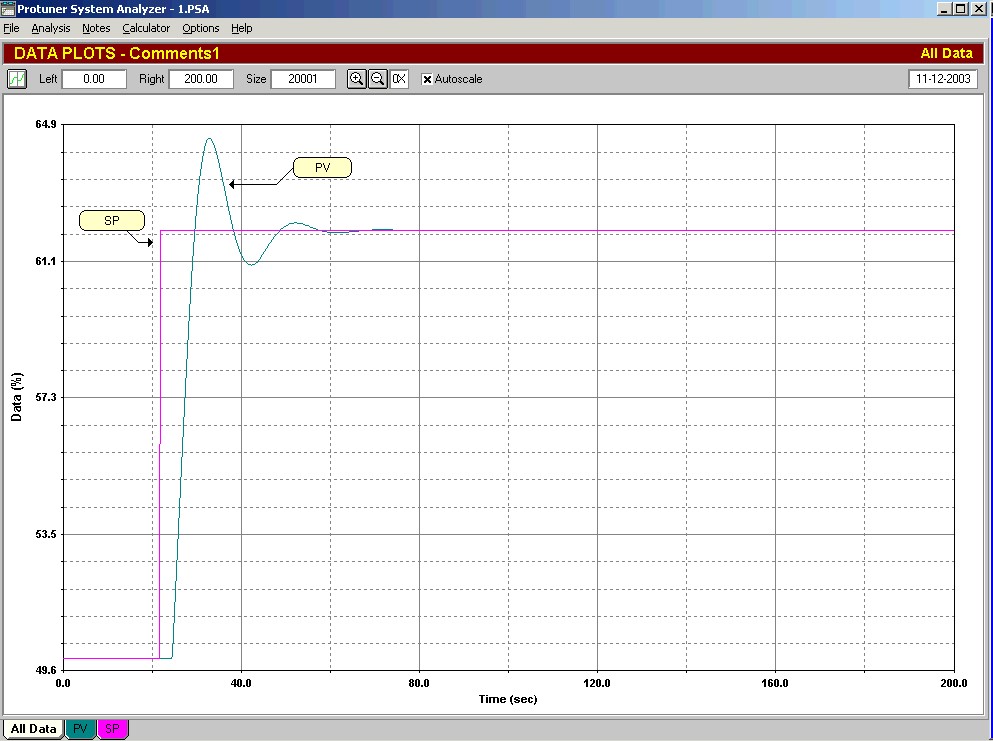
Figure 4 is the Cohen Coen result. It is terrible, and is in fact pretty close to instability. A very dangerous tune.
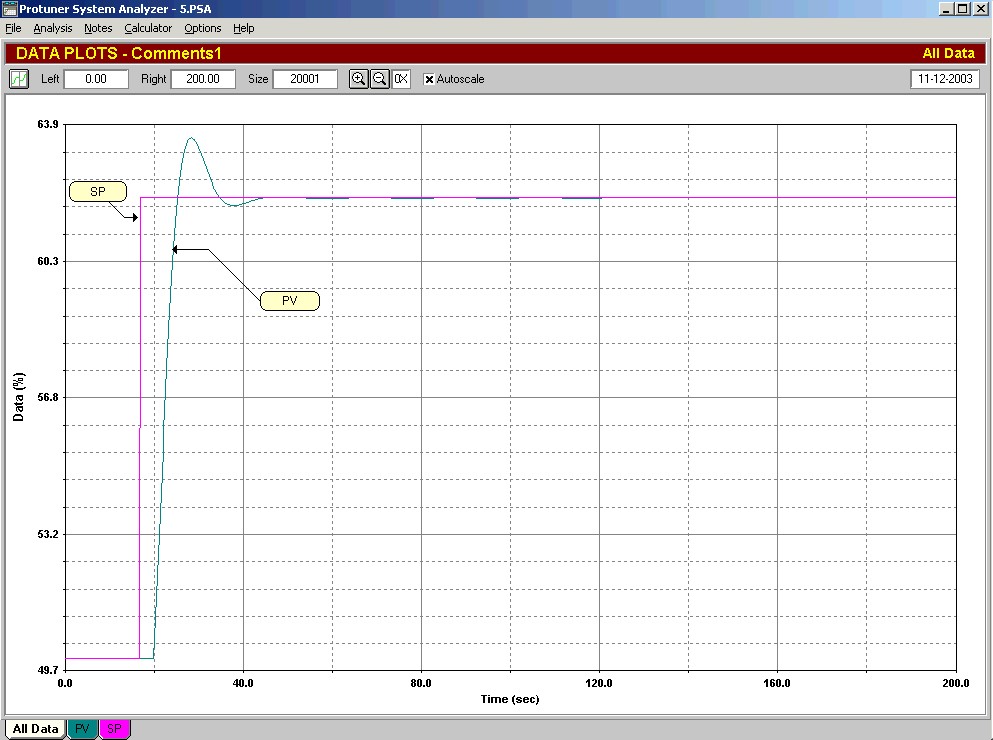
Figure 5
Figure 5 is the Protuner tuning, and gives a result exactly what it is supposed to have given, namely one overshoot and one undershoot. This is not surprising. The method uses all the theory and more. As I often mention to course delegates, if it wouldn’t be worth much if it couldn’t tune a terribly simple process like this. It’s a bit like using a sledgehammer to crack a nut.
I also believe that this example shows also shows that if all the other SWAG methods have difficulties tuning a simple process like this, then there is little chance of them succeeding when one deals with more difficult process dynamics, and justifies my contention that in fact you do need a sophisticated scientifically based tuning package like that in the Protuner if you are serious about optimisation.
In the next article in this series, which will be published in two months time, using the results obtained from the tuning above, we will investigate the basics of tuning theory, and try and gain an understanding of some of the really important principles which one should understand about tuning self-regulating processes.
I would recommend that you keep the previous articles and this one handy, as you will need to refer to them again.